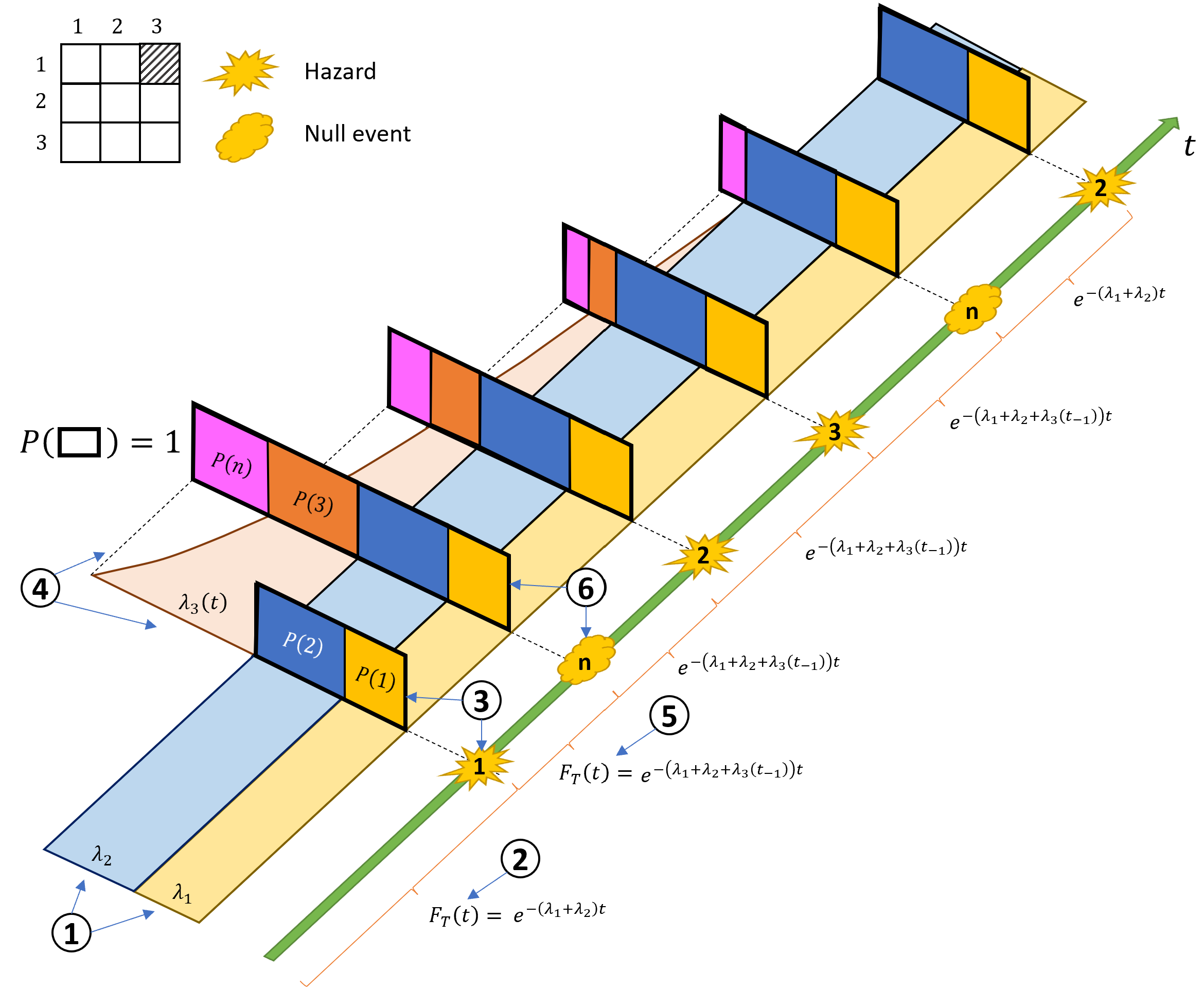
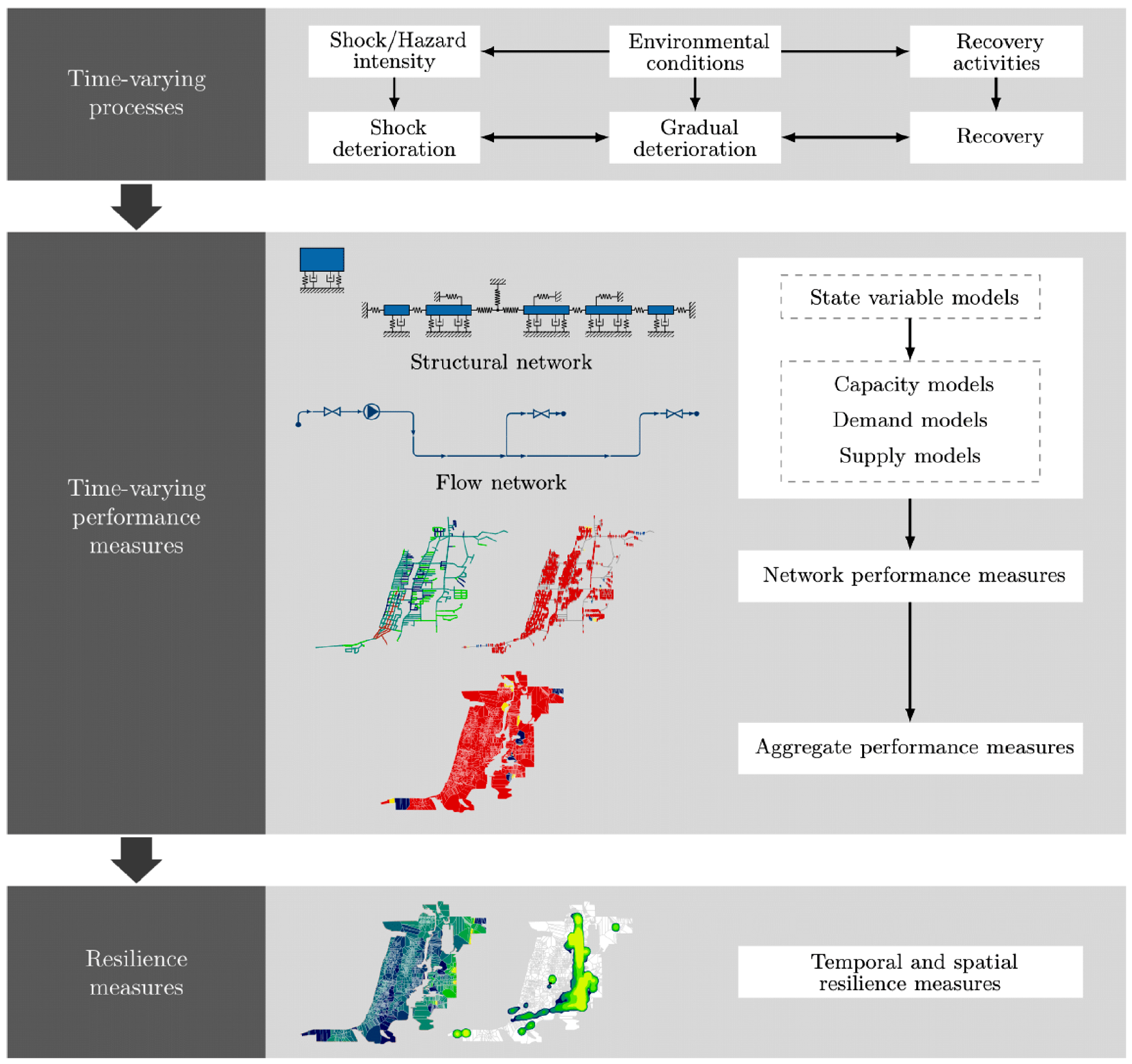
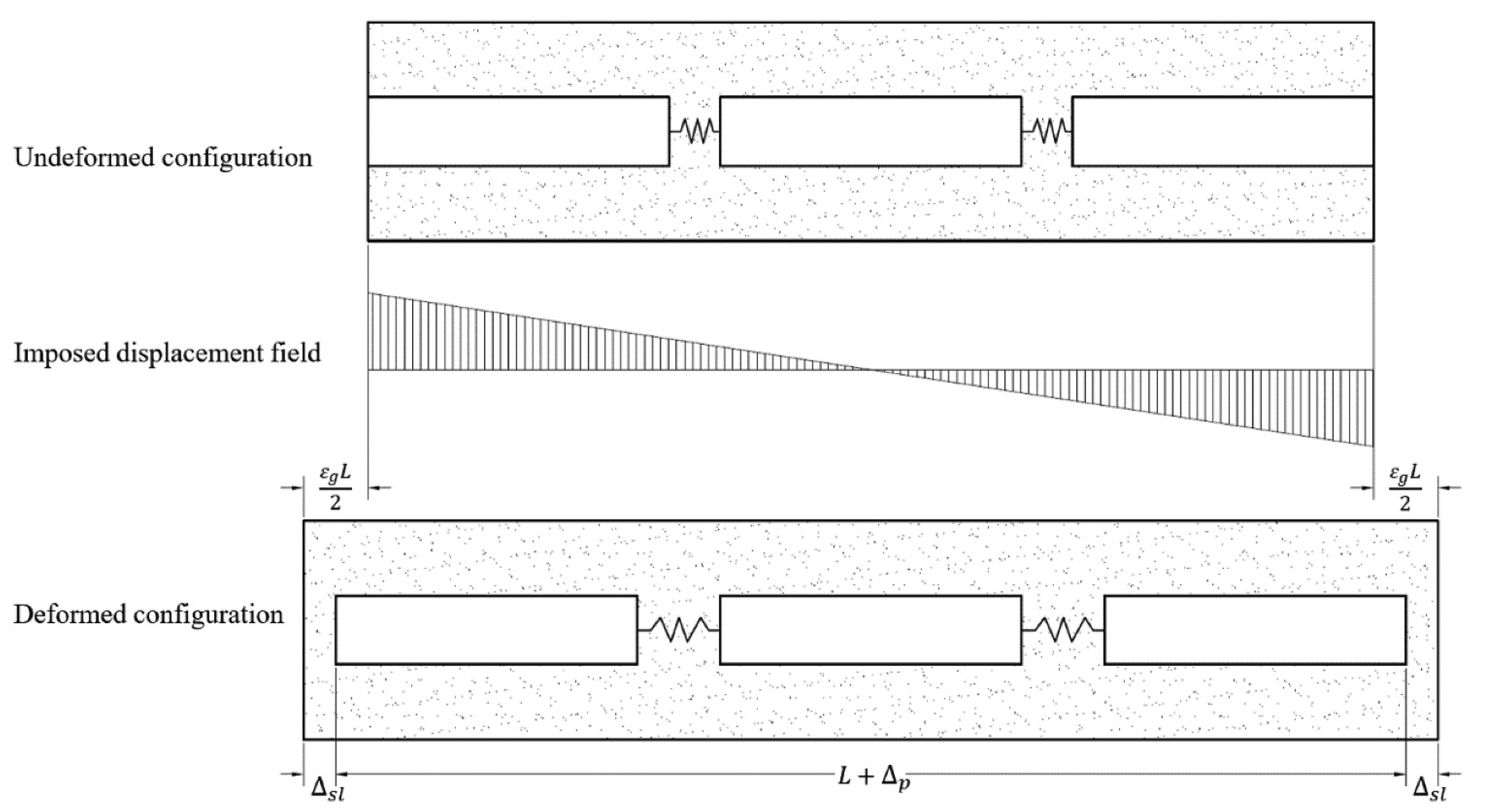
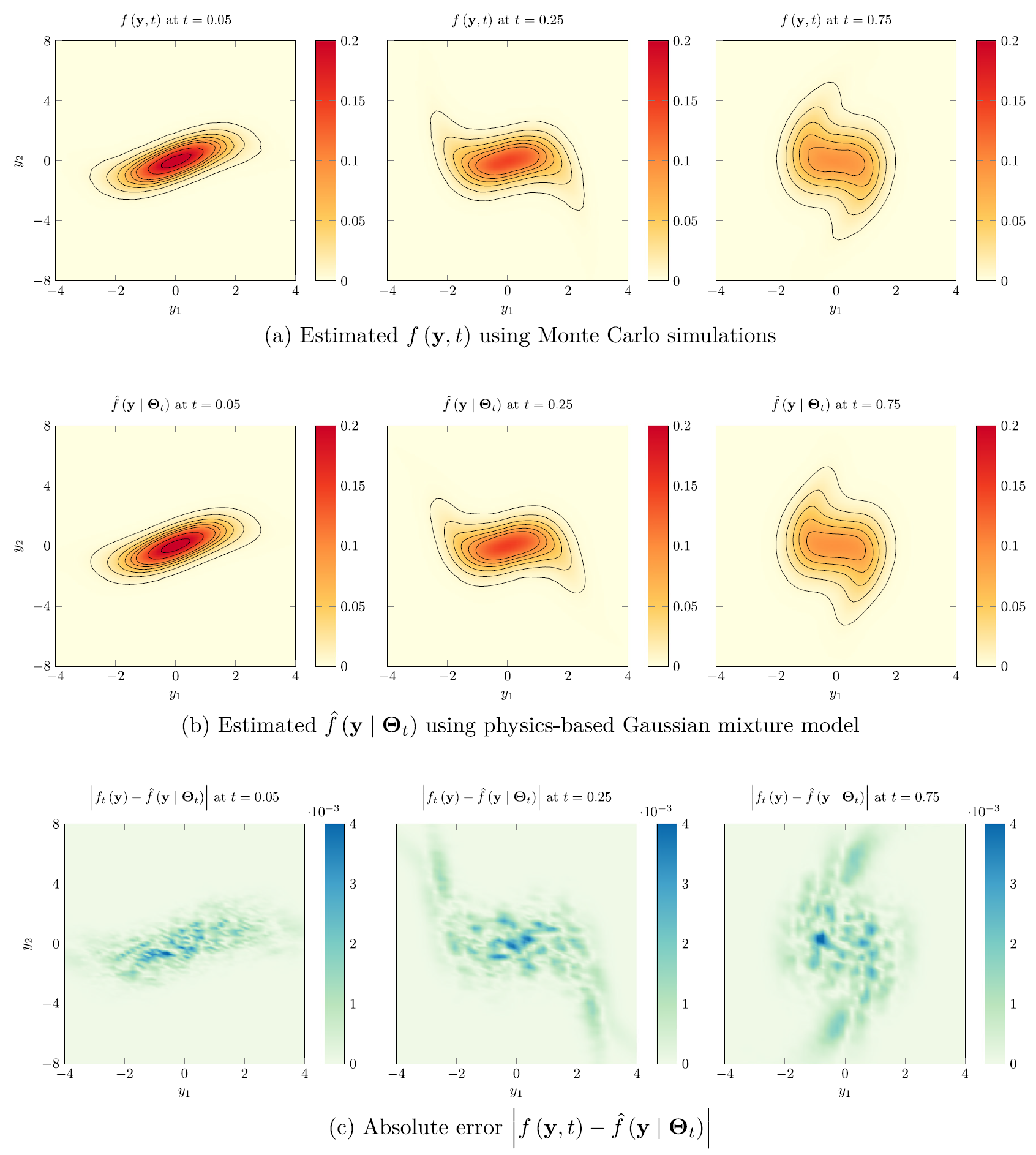
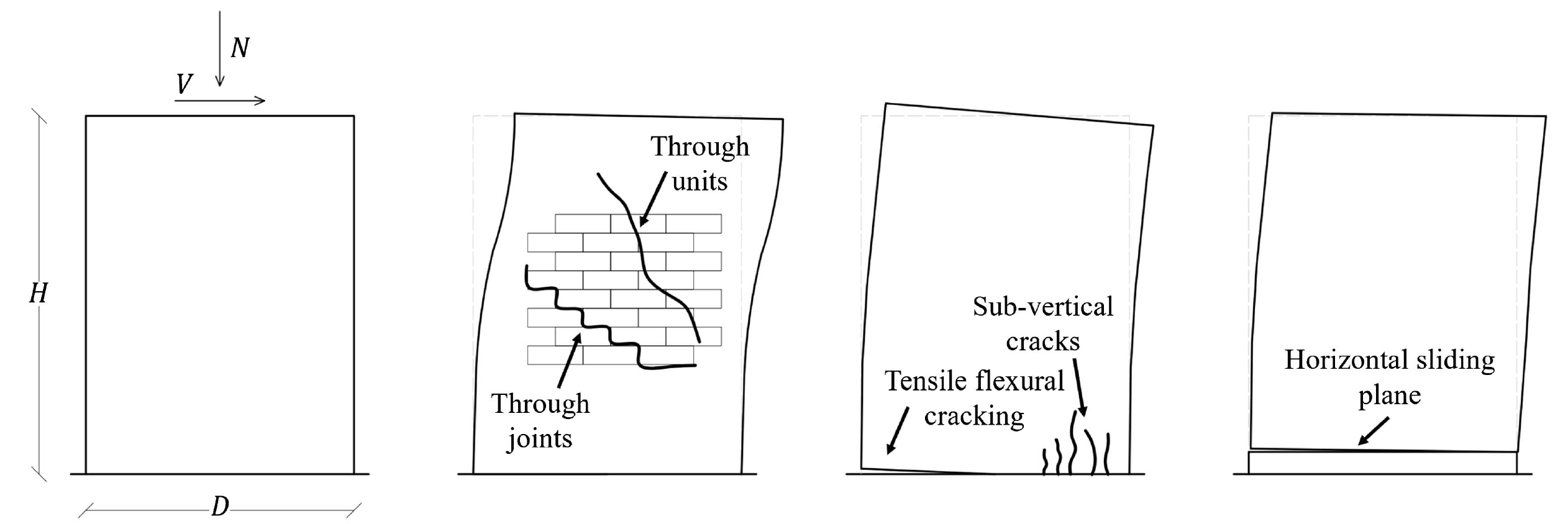
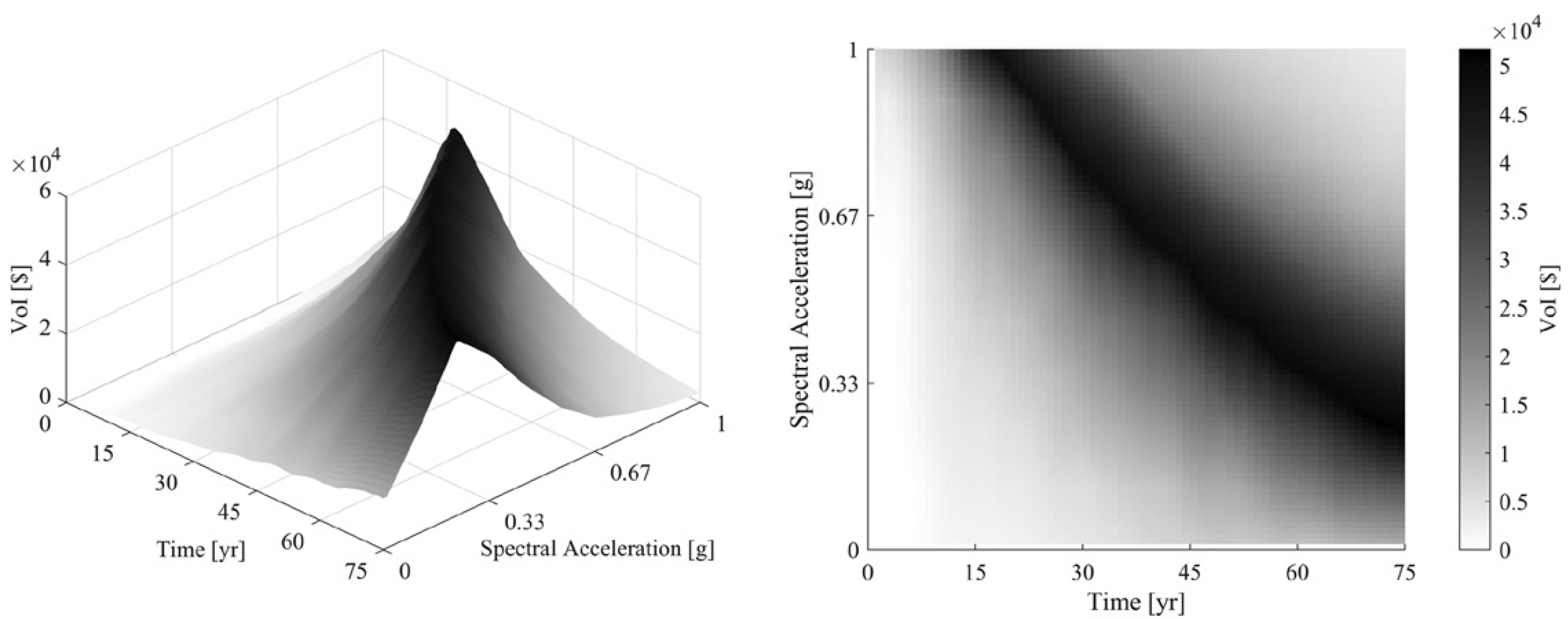
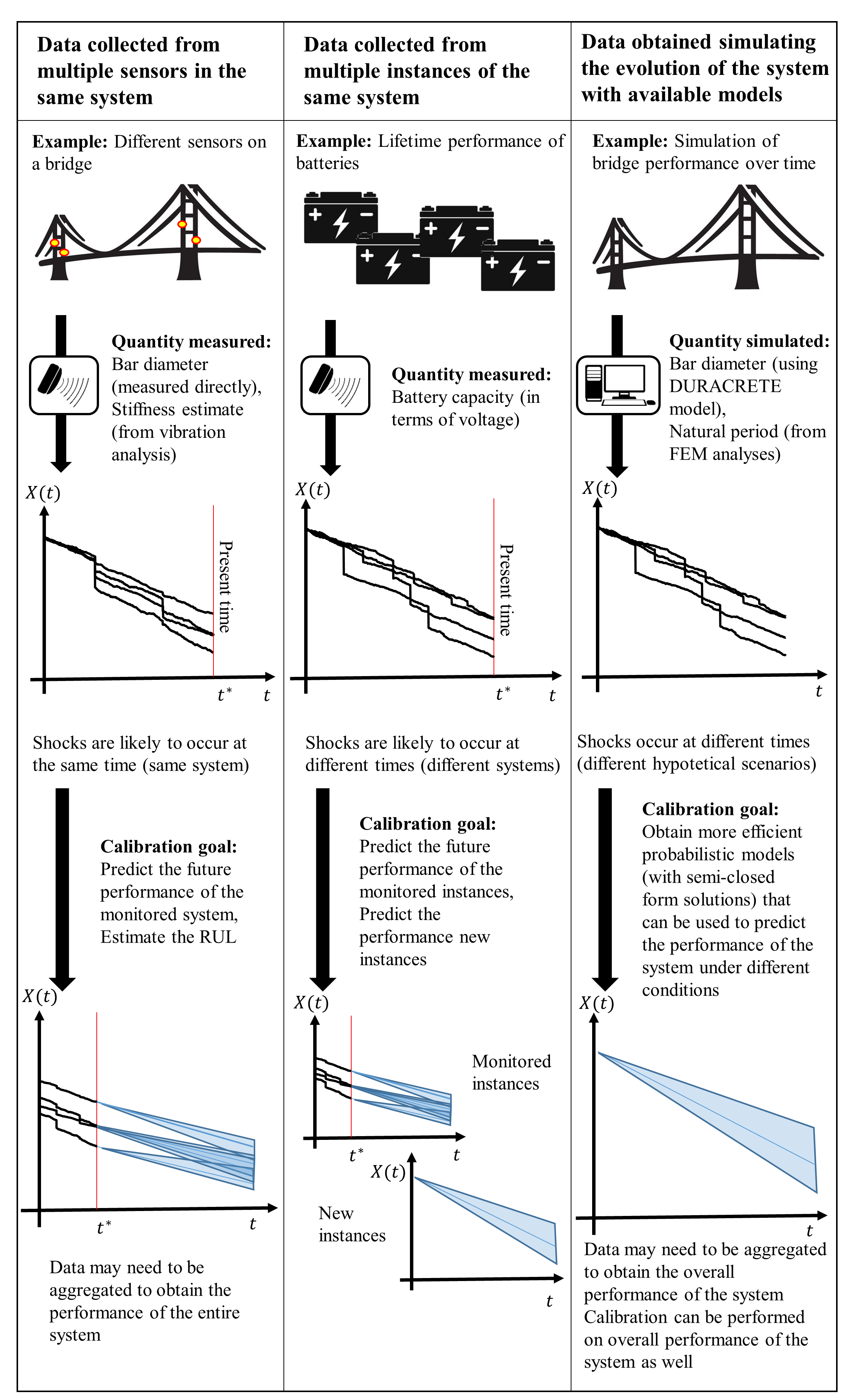
Understanding, modelling, and reducing time-varying risk induced by natural hazards on vulnerable communities

In my research, I passionately employ cutting-edge statistical techniques to unravel the dynamic evolution of infrastructure and the built environment. By delving deep into the analyses, we gain invaluable insights that steer us towards a brighter and more sustainable future.

Simulating interacting multiple natural hazard events for lifecycle consequence analysis

Among different types of natural-hazard interactions, some occur through the nature of the hazards themselves, regardless of the presence of any physical assets: they are often called “Level I” (or occurrence) interactions. In such cases, one hazard event triggers or modifies the occurrence of another (e.g., severe wind and flooding; liquefaction and landslides triggered by an earthquake), thus creating a dependency between the parameters characterising such hazard events. They differ from “Level II” (or consequence) interactions, which instead occur through impacts/consequences on physical assets/components and systems. Multi-hazard Life Cycle Analysis (LCA) aims to quantify the consequences (e.g., repair costs, downtime, and casualty rates) expected throughout a system’s service life, accounting for Level I and II interactions. Nevertheless, the available literature generally considers these interactions mainly defining relevant taxonomies, often qualitatively, without providing a computational framework to simulate a sequence of hazard events in terms of their occurrence times and features and resulting consequences. This work aims to partly fill this gap by identifying modelling approaches associated with different Level I interactions. It describes a simulation-based approach for generating multi-hazard scenarios (i.e., a sequence of hazard events and associated features through the system’s life cycle) based on the theory of competing Poisson processes. The proposed approach incorporates the different types of interactions in a sequential Monte Carlo sampling method. The method outputs potential sequences of events throughout a system’s life cycle, which can be integrated into LCA frameworks to quantify interacting hazard consequences.

Collaborators: Roberto Gentile and Carmine Galasso (UCL)

Simulation-based flood fragility and vulnerability analysis for expanding cities

Accurately quantifying flood-induced impacts on buildings and other infrastructure systems is essential for risk-sensitive planning and decision-making in expanding urban regions. Flood-induced impacts are directly related to the physical components of assets damaged due to contact with water. Such components include building contents (e.g., appliances, furniture) and other non-structural components whose damage/unavailability can severely impact the buildings’ functionality. Conventional fragility analysis approaches for flooding do not account for the physical damage to the individual components, mostly relying on empirical methods based on historical data. However, recent studies proposed simulation-based, assembly-based fragility models that account for the damage to the building components. Such fragility models require developing detailed inventories of vulnerable components of households and identifying building archetypes to be considered in a building portfolio for the region of interest. Content inventories and building portfolios have so far been obtained for specific socio-economic contexts such as the United States of America. However, building types and their content can significantly differ between countries, making the available fragility models and computational frameworks unsuitable for flood vulnerability analysis in rapidly expanding cities characterised by extensive informal settlements, such as low- and middle-income countries. The aim of this work is to detail how to adapt the available methodologies for flood vulnerability assessment to the context of formal and informal settlements of expanding cities in the global south. It also details the development of content inventories for households in these cities using field surveys. The proposed survey is deployed in various areas vulnerable to floods in Kathmandu, Nepal. Based on the survey results, each component within the household is associated with a corresponding flood capacity (resistance) distribution (in terms of water height and flood duration). These distributions are then employed in a simulation-based probabilistic framework to obtain fragility relationship and consequence models. The relevant differences between the results obtained in this study and those from previous studies are then investigated for a case-study building type. In addition, the influence of socio-economic factors (e.g., household income) and past flood experience (possibly resulting in various flood-risk mitigation strategies at a household level) on the resulting flood impacts is also included in the model.

Collaborators: Vibek Manandhar (NSET), Januka Gyawali (Practical Action), Prayash Malla (NSET), Roberto Gentile (UCL), Maggie Creed (University of Glasgow), Ramesh Guragain (NSET), and Carmine Galasso (UCL)

Modelling Time-varying Reliability and Resilience of Deteriorating Infrastructure

In this work, we propose a general formulation to model the physical state and functionality of deteriorating infrastructure throughout its service life. The paper unifies physics-based models for deterioration and recovery. It also develops resilience metrics to quantify the temporal and spatial variations of the infrastructure’s ability to recover after disruptive events. These metrics can assess the disparity in the recovery process across various sections of the infrastructure. The practical application of this framework is demonstrated through a case study on the water infrastructure of a coastal community in Oregon, USA.

Collaborators: Armin Tabandeh, Neetesh Sharma and Paolo Gardoni (UIUC)

Paper

Physics-based Repair Rate Curves for Segmented Pipelines subject to Seismic Excitation

In this work we develop probabilistic physics-based Repair Rate (RR) curves to quantify the damage to segmented pipelines due to seismic excitations. First, a mechanical model for segmented pipelines is proposed. Then, the model is used to generate a set of synthetic data to calibrate the model parameters of the developed probabilistic formulation. The proposed RR curves are compared with the ones available in the literature, and the advantages of the proposed formulation are discussed.

Collaborator: Paolo Gardoni (UIUC)

Paper

Numerical Solution of the Fokker-Planck Equation using Physics-based Mixture Models

In this work we develop a novel numerical method based on physics-based mixture models for the transient and steady-state solutions of the Fokker–Planck equation. The unknown parameters of the mixture model are estimated via Bayesian inference while considering the physical constraints on their numerical value. The proposed method can be used to efficiently estimate the time-varying distribution of variables that evolve according to processes described by stochastic differential equations.

Collaborators: Armin Tabandeh, Neetesh Sharma and Paolo Gardoni (UIUC)

Paper

Derailment simulation analysis of the relationship between railroad tank car structural modeling, physical testing, and the probability of damage and release

In this work we developed an innovative analytical framework to predict the capacity of novel tank car designs to resist puncturing forces during derailments. A finite element model is developed to generate impact forces on railcars after the initiation of train derailments. Such forces are then integrated within a probabilistic framework to predict the probability of puncture and release of hazardous materials into the environment.

Collaborators: Steven W. Kirkpatrick (ARA), Chen-Yu Lin (UIUC), Paul Gharzouzi (UIUC), Todd Treichel (RSI-AAR), Christopher P.L. Barkan (UIUC) and Paolo Gardoni (UIUC)

Paper

Probabilistic Models and Fragility Estimates for Hollow Clay Bricks’ URM Walls subject to In-plane Horizontal Forces

In this work we develop probabilistic capacity models for unreinforced masonry walls with hollow clay units subject to horizontal in-plane forces. The models are developed considering diagonal cracking, flexural/rocking, and sliding failure as possible failure modes. They are constructed from existing physics-based models that attempt to capture the underlying physics, then integrated with correction terms that improve their accuracy and remove the inherent bias. Unknown parameters for the proposed models are calibrated using a Bayesian updating approach.

Collaborators: Marco Andreini (CERN), Mauro Sassu (University of Pisa) and Paolo Gardoni (UIUC)

Paper

Quantifying the Value of Information of Multiple Inspection Methods for Degrading Engineering Systems

In this work we propose a formulation to compute the Value of Information (VoI) of inspections and structural health monitoring for degrading engineering systems. In the proposed formulation, the information collected is used to improve the prediction of the effects of gradual and shock deterioration processes and the future probability of failure. The results of the VoI analysis can be used to evaluate whether it is convenient to install monitoring and/or perform inspections on a given system (such as a bridge) after a disastrous event.

Collaborators: Pier Francesco Giordano , Maria Pina Limongelli (Politecnico di Milano) and Paolo Gardoni (UIUC)

Paper

Stochastic Differential Equations for the Deterioration Processes of Engineering Systems

In this work we propose to use a system of Stochastic Differential Equations to model the evolution of the physical properties of an engineering system over time. The proposed formulation captures the continuous nature of the degradation processes acting on the system and considers their possible interactions. In addition, results from stochastic calculus are used to facilitate the simulation of the processes and to obtain closed-form solutions for the distribution of the physical properties over time. An extended version of this conference paper has been submitted to the Journal for Reliability Engineering and System Safety and is currently under review.

Collaborator: Paolo Gardoni (UIUC)